23.1 Electric Potential Energy and Difference #

• PE can only be defined for conservative forces
  • That is, work done by said force is independent of the path taken
  • Coulomb’s Law is conservative because the dependence on position is conservative
• Hence, we define .$\Delta U = -W$ with
  • .$\Delta U = U_b - U_a$ is for a situation where a point charge .$q$ moves from point .$a$ to point .$b$
  • This is equal to negative work, .$-W = -\vec F d = -(q\vec E) d$ (for a uniform .$\vec E$)

23.2 Relation between Electric Potential and Field #

• Electric Potential: Electric PE per unit charge, such as for a charge at point .$a$ $$V_a = \frac{U_a}{q}$$
• We only really care about difference though, which is defined as $$V_{ba} = \Delta V = \frac{U_b - U_a}{q} = - \frac{W_{ba}}{q}$$
• We can now also define PE in terms of electric potential: $$\Delta U = U_b - U_a = q(V_b - V_a) = qV_{ba}$$
• Electric potential difference is a measure of how much energy an electric charge can acquire in a given situation.
• Since energy is the ability to do work, the electric potential difference is also a measure of how much work a given charge can do.
  • The exact amount of energy or work depends both on the potential difference and on the charge.
• If a positive charge is free, it will tend to move from high to low potential
  • Inverse for opposite charge

23.3 Potential due to Point Charges #

$$\Delta U = U_b - U_a = - \int_a^b \vec F \cdot d \vec l$$

• .$dl$ is an infinitesimal increment of displacement along the path from .$a$ to .$b$
  • Keep in mind that .$\vec F$ must be conservative
  • Thus the integral can be taken along any path from point .$a$ to point .$b$.
• Knowing .$\vec E = \vec F / q$ and .$V_{ba} = (U_b - U_a) / q$, we can write the electric potential equation as…
  

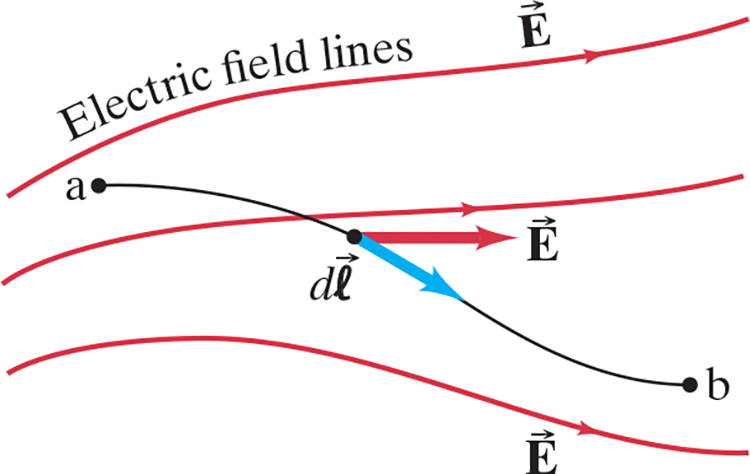
  $$V_{ba} = V_b - V_a = - \int_a^b \vec E \cdot d \vec l$$ $$V_{ba, \text{uniform $\vec E$}} = -E\int_a^b d\vec l = -Ed$$ …where .$d$ is the distance of a straight line from point .$a$ to .$b$

Charged Conducting Sphere #

1. Electric Potential Outside Sphere #

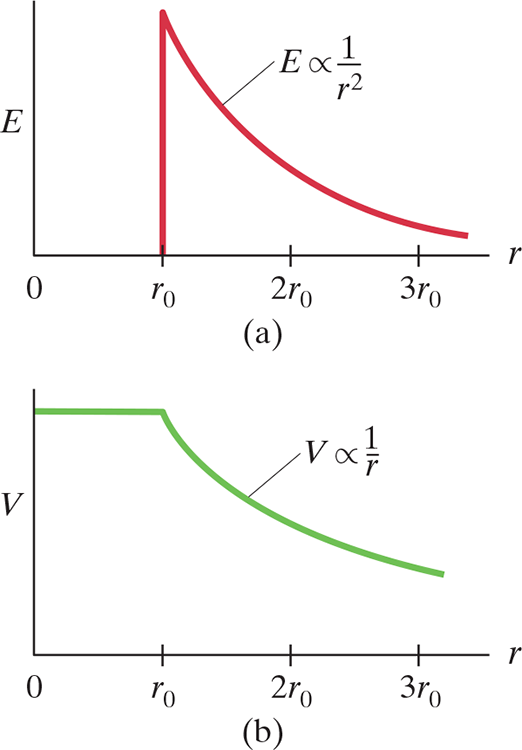
• We know .$\vec E = \frac{1}{4\pi\varepsilon_0} \frac{Q}{r^2}$ for outside a conducting sphere (.$r > r_0$)
• Therefore, we can write $$V_{ba} = - \int_{r_a}^{r_b} \vec E \cdot d \vec l = - \frac{Q}{4\pi\varepsilon_0}\int_{r_a}^{r_b} \frac{dr}{r^2}$$ $$\dots = \frac{Q}{4\pi\varepsilon_0} \bigg(\frac{1}{r_b} - \frac{1}{r_a}\bigg)$$ $$\dots = \frac{1}{4\pi\varepsilon_0} \frac{Q}{r} \text{ [$r_b = \infty$]}$$

• Thus, the whole conductor, not just its surface, is at this same potential.
• We can also generalize the first case to the electric potential .$r$ from a single point charge .$Q$

Coulomb potential #

• The potential outside a uniformly charged sphere is the same as if all the charge were concentrated at its center

  The potential near a positive charge is large, and it decreases toward zero at very large distances

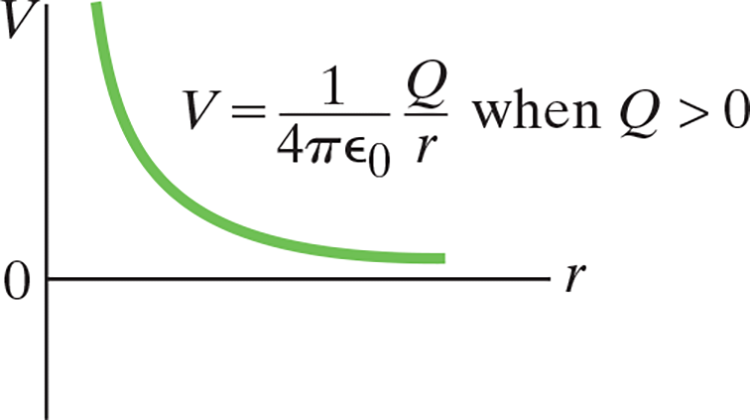
  For a negative charge, the potential is negative and increases toward zero at large distances

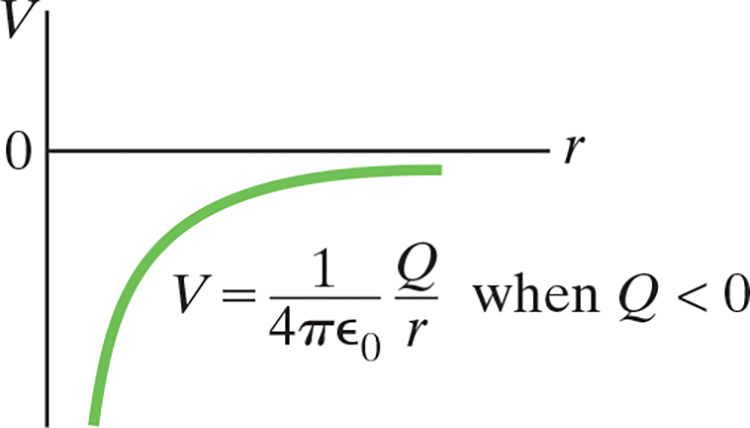
23.4 Potential due to Any Charge Distribution #

• If .$\vec E$ is a function of position (or otherwise unknown), we can find .$V$ by calculating the potential due to the many tiny charges that make up .$\vec E$: $$V = \frac{1}{4\pi\varepsilon_0} \int \frac{dq}{r}$$ where .$r$ is the distance from a tiny element of charge .$dq$ to the point where .$V$ is being determined

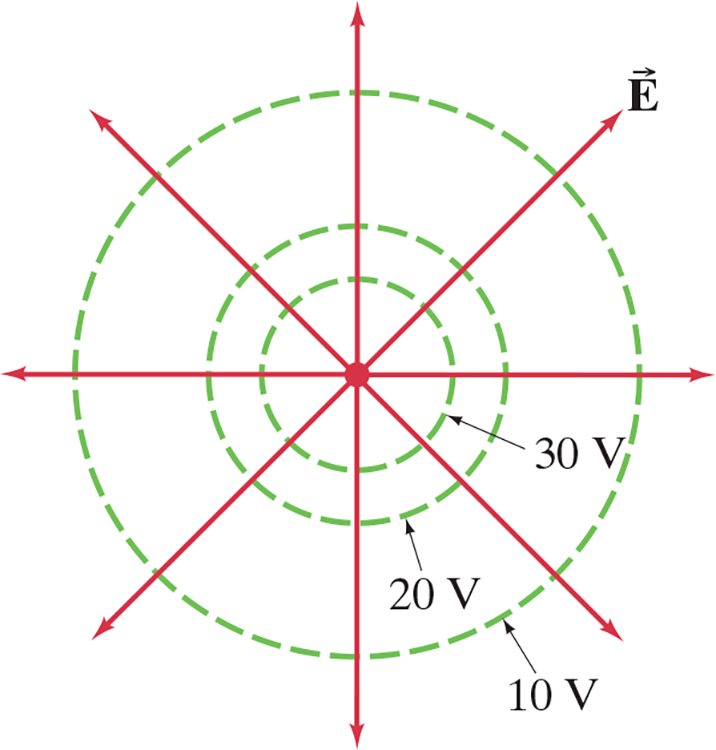
23.5 Equipotential Lines and Surfaces #

• The electric potential can be represented by drawing equipotential lines, or, in three dimensions, equipotential surfaces
• An equipotential surface has all points at the same potential.
  • That is, the potential difference between any two points on the surface is zero
  • Thus, no work is required to move a charge from one point on the surface to another.
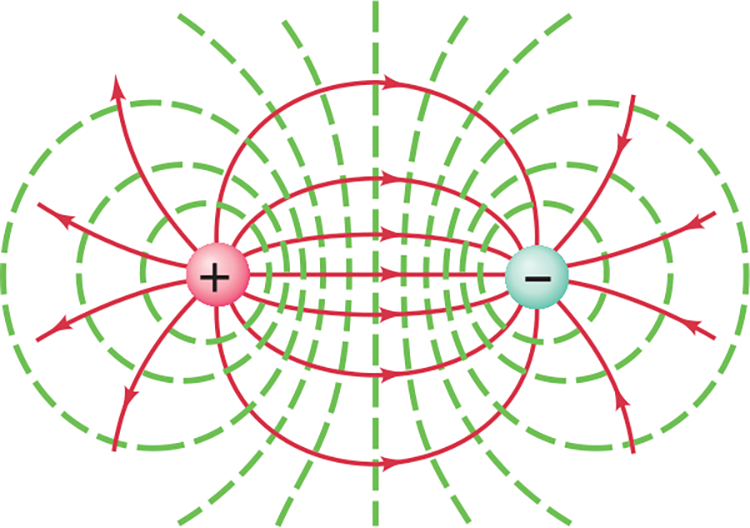
• Equipotential surfaces are perpendicular to the electric field (field lines)
• For a positive point charge, the equipotential surface with the largest potential is closest to the positive charge
• Unlike electric field lines, which start and end on electric charges, equipotential lines/surfaces are always continuous and never end

23.6 Potential Due to Dipole (Moment) #

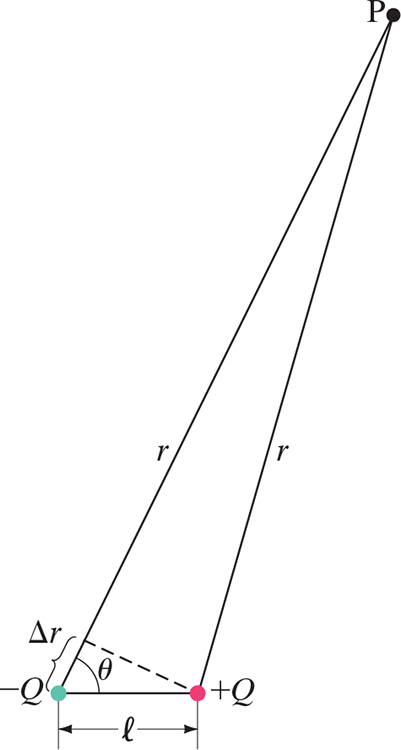
$$V = \frac{1}{4\pi\varepsilon_0} \frac{Q}{r} + \frac{1}{4\pi\varepsilon_0} \frac{(-Q)}{(r+\Delta r)} = \frac{Q}{4\pi\varepsilon_0} \frac{\Delta r}{r(r + \Delta r)}$$

• .$r$ is the distance from (some arbitrary point) .$P$ to the positive charge and .$r + \Delta r$ is the distance to the negative charge
  • If .$r \gg l$, then .$r \gg \Delta r \approx l \cos \theta$ so we can neglect .$\Delta r$ $$V = \frac{1}{4\pi\varepsilon_0} \frac{Ql \cos\theta}{r^2} = \frac{1}{4\pi\varepsilon_0} \frac{p \cos\theta}{r^2} $$
  • Notice the potential decreases .$\propto r^2$, whereas for a single point charge the potential decreases .$\propto r$
  • It is not surprising that the potential should fall off faster for a dipole:
    • When you are far from a dipole, the two equal but opposite charges appear so close together as to tend to neutralize each other

  

23.7 .$\vec E$ Determined from .$V$ #

• We know that .$V_b - V_a = - \int_a^b \vec E \cdot d\vec l$, which we can write in differential form as .$dV = -\vec E \cdot d\vec l = - E_l dl$. This can be written as $$E_l = - \frac{dV}{dl}$$
• .$dV$ is the tiny difference in potential between two points a distance .$dl$ apart, and .$E_l$ is the component of the electric field in the direction of the tiny displacement .$d\vec l$
• This is called the gradient of .$V$ in a particular direction: The general case is $$\vec E = - \nabla \vec V = - \bigg\langle \frac{\delta V}{\delta x}, \frac{\delta V}{\delta y}, \frac{\delta V}{\delta z} \bigg\rangle$$
• This states that the electric field points “downhill” towards lower voltages (where there is lower potential)

23.8 Electrostatic PE; The Electron Volt #

• The electric potential and energy potential due to one point charge .$Q_1$ on another point charge .$Q_2$ separated by .$r_{12}$ are $$V = \frac{1}{4\pi\varepsilon_0} \frac{Q_1}{r_{12}}$$ $$U = Q_2 V = \frac{1}{4\pi\varepsilon_0} \frac{Q_1 Q_2}{r_{12}}$$
• The PE is the negative work needed to separate the two charges to infinity.
• For three points, we can use the superposition principle like we have prior to write $$U = \frac{1}{4\pi\varepsilon_0}\bigg( \frac{Q_1 Q_2}{r_{12}}+ \frac{Q_1 Q_3}{r_{13}} + \frac{Q_2 Q_3}{r_{23}} \bigg)$$

Electron Volt #

• Joules are a very large unit for dealing with energy of the electron scale; as such, the electron volt (.$eV$) is often used
• One electron volt is the energy acquired by a particle carrying a charge .$e$ (the magnitude of an electron) as a result of moving through a potential difference of .$1 V$ $$1 \text{ eV} = 1.6022 \cdot 10^{-19} \text{ J}$$
• E.x., an electron (charge .$e = 1.6\cdot10^{-19}$) that accelerates through a potential difference of .$1000 \text{ V}$ will lose .$1000 \text{ eV}$ of potential energy and gain .$1000 \text{ eV}$ of kinetic energy

23.9 Digital; Binary Numbers; Signal Voltage (not covered) #

• Batteries and wall sockets provide a steady supply voltage as power
• Signal voltage provide/carry information
  • Analog signal voltage has voltage that varies continuously (i.e .$\sin$)
  • Digital signals are more complicated and encode information, often in binary
    • Bytes have 8 bits which allow .$2^8 = 256$ numbers
    • Digital signals are transmitted at some rate (bit-rate) given in .$\text{Mb/s}$

• Digital Signals
  • Digital to Analog, DACs, exist too because some appliances require an analog signal
  • Digital signals can be compressed: Repeated information can be reduced so that less memory (bits) is needed
    • Fun fact: Bit is the contraction of “binary digit”, leaving out the 8 letters between
  • Digital signals are more resistant from noise, which badly corrupts analog signals
    • Any electronic signal involves electric charges whose electric field can affect charges in another nearby signal
    • External fields, as from high voltage wires, motors, or fluorescent lamps, can produce noise
    • Thermal noise refers to random motion of electrons, much like the “thermal motion” of the molecules in a gas
    • Moving electrons can be affected by the medium (wire, etc.), altering the signal